Laser-driven ion acceleration can generate mono-energetic ions with characteristics suitable for a variety of applications. Important examples are proton therapy for treatment of deep tumors in cancer patients, and the fast ignition scheme of inertial confinement fusion. In both cases the advantage of using ions, often protons, is their ability to deposit their kinetic energy in a small target volume, known as the Bragg peak. Laser-plasma interactions can also support accelerating fields orders of magnitude higher than conventional accelerators and produce beams of much higher intensity.
Acceleration of ions is accomplished with an ultraintense laser incident on an ultrathin foil, which is instantly ionized into an overdense plasma. In the radiation pressure acceleration (RPA) regime, electrons in the foil are pushed via the laser ponderomotive force, and set up the electrostatic acceleration field, which accelerates the ions. Circularly polarized lasers prevent electron heating, as the ponderomotive force is constant in time.
There are key technologically and fundamentally important questions remaining for RPA. One of such is the Classical Rayleigh-Taylor Instability (RTI) which occurs when a light fluid(photon) accelerates a heavy fluid (plasma ions). Ripples are generated on the over-dense plasma foil surface, leading to degradation of acceleration process, transverse density modulation, and even termination of the acceleration process.
Our group has pioneered a variety of methods to model the acceleration process and curtail the instability.
We have developed a self-consistent kinetic theory of a laser-accelerated plasma target with distributed electron/ion. We identified and analytically described several distinct target regions (nonneutral ion tail, non-neutral electron sheath, and neutral plasma bulk) in the target uniformly accelerated by the self-consistent electric field generated by hot electrons trapped in combined ponderomotive and electrostatic potentials moving. Using this model, we have recovered the classical RTI growth rate, but also have found that growth rate is reduced in some regimes.

Fig 1. Kinetic model of laser-accelerated plasma target
We also discovered that a hydrocarbon foil can be used to stabilize the acceleration process; under this scheme, the lighter protons move ahead of the heavier carbon ions. The buffer zone formed by the carbon ions protect the protons from the unstable interface.

Fig 2. Stability of multispecies target and single-specie target.
Recently, we have discovered that an aptly designed thin target can simultaneously get accelerated and focused to a singular spot. This scheme is a twist on light-matter interaction. In optics, matter’s refractive index focuses light, but the converse is also true; an intense flux of photons, i.e. laser, can do the same for matter, i.e. overdense plasma. Hence, the scheme is named Laser-Ion-Lens Accelerator (LILA).
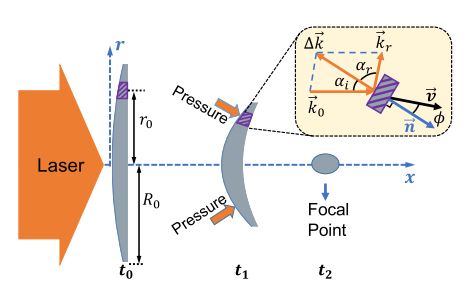
Fig 3. Schematic of Laser-Ion-Lens Accelerator
It was observed that the convergent plasma flow can, under appropriate physical conditions, merge the density ripples and suppress RTI growth. Furthermore, the plasma density increase during the focusing process keeps the plasma overdense despite the heating, producing a GeV-scale monoenergetic peak in the energy spectrum.
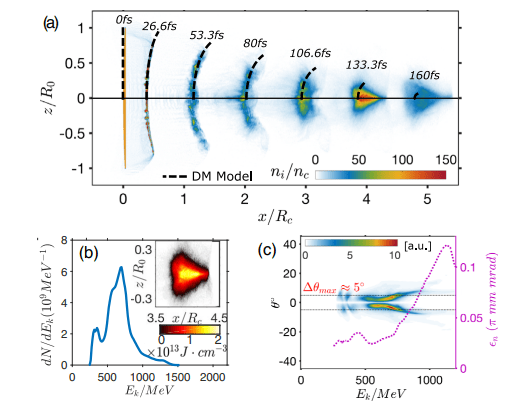
Fig 4. Simulation result for Laser-Ion Lens Accelerator. (a) The initially flat target undergoes bending and focusing during the interaction, forming a singularly focused relativistic plasma beam at 133.3fs. (b) The plasma beam has a monoenergetic energy peak and ultra-high energy density at focus. (c) The resulting ion beam is well-collimated and has very low emittance.
We are currently exploring advanced ion acceleration schemes that can overcome the transverse instability, enabling RTI-stable ion accelerators that are amenable to different laser polarizations, multispecies targets, target geometry, and ion energy ranges.
Relevant Publications:
•T. P. Yu, A. Pukhov, G. Shvets, and M. Chen, “Stable Laser-Driven Proton Beam Acceleration from a Two-Ion-Species Ultrathin Foil,” Phys. Rev. Lett. 105, 065002 (2010).
• V. Khudik, S. A. Yi, C. Siemon, and G. Shvets, “The analytic model of a laser-accelerated plasma target and its stability”, Phys. Plasmas 21, 013110 (2014).
• T. Wang, V. Khudik, G. Shvets, “Laser-Ion Lens and Accelerator”, Phys. Rev. Lett. 126, 024801(2021)
